A Design System for Orthogonal Pleat Tessellation

Abstract

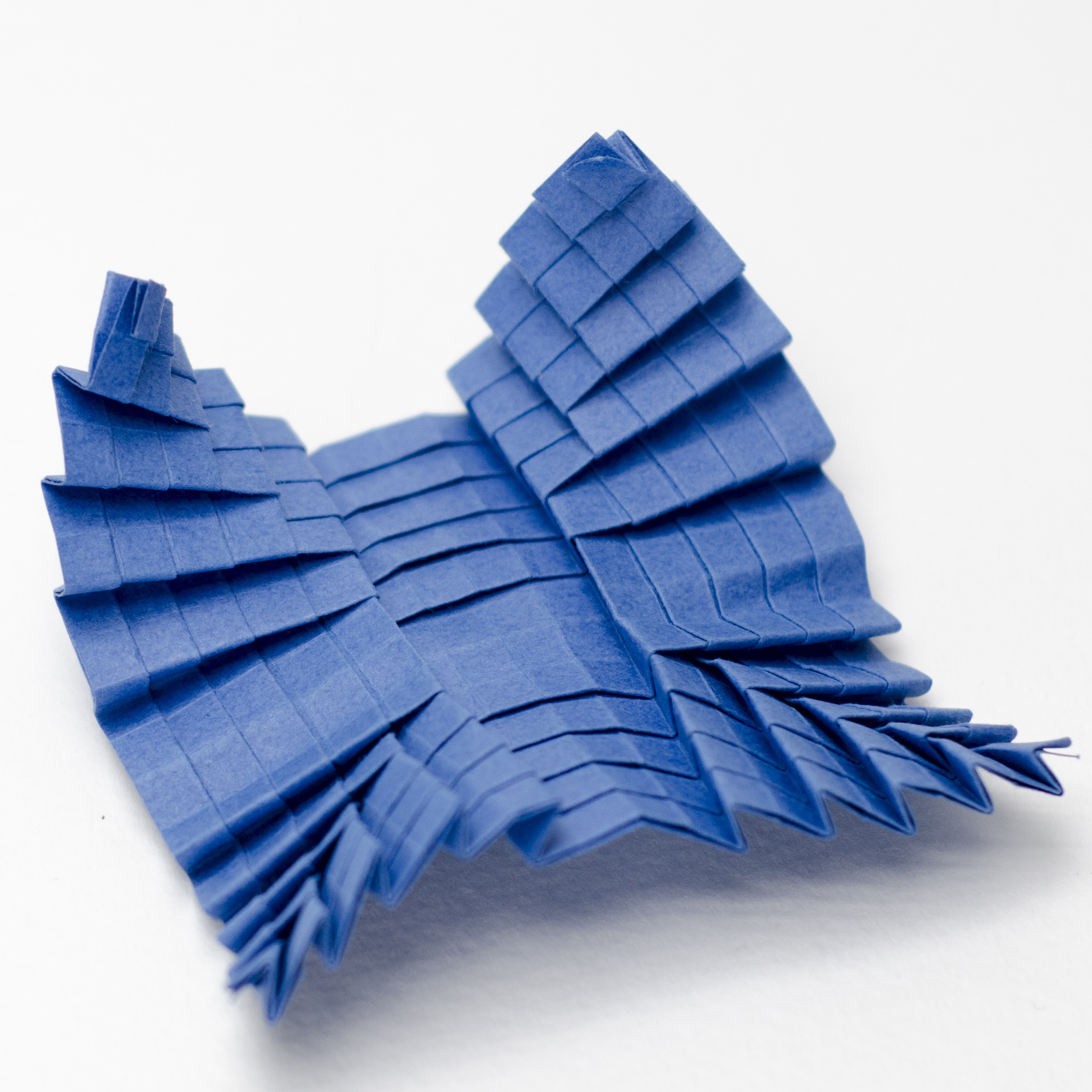
Pleat tessellation is a simple origami tessellation that contains only pleat folds. A pleat fold is formed by a pair of parallel mountain fold and valley fold. Despite its simplicity, pleat tessellation has its own advantages such as the easy generation of three-dimensional shapes. In this paper, we study a very common form of pleat tessellations that contains only orthogonal pleats. For this particular kind of pattern, we want to simplify the universal origami theorems and to develop better algorithms for computing the folding sequences. We enumerate all its eight basic units which appear in crease patterns of orthogonal pleat tessellations and propose a new notation to rewrite the crease pattern. With this notation, users can easily input height information to design desired shapes. Although the definition of orthogonal pleat tessellation is very straightforward, it is not easy to fold it form the crease pattern. We propose a method to compute the folding sequence, normally not unique, from its crease pattern. In addition, based on this notation we also notice the existence of a combination of pleat units, which is referenced here as cyclic pleat pattern. We describe the characteristics of the cyclic pleat pattern and propose an algorithm to detect it in its crease pattern. Finally, we introduce a system that, as input, receives a matrix of our notations.

Works

Application